

Let's look at the examples given in the examples section for better clarity. Step 3: If the above conditions are satisfied, the given triangles are considered congruent by the ASA rule.Step 2: Make comparisons if two angles with one included side of a triangle are equal to the corresponding two angles and included side of the other triangle.Step 1: Look at the triangles and check for the given angles and sides.To apply the ASA congruence rule for any two given triangles, follow the steps as given below: In order to check if the given triangles are congruent, the best way is to superimpose them, put them one over the other. We may even call it the AAS Congruence Rule. Therefore, we can conclude that the two triangles are congruent if any two pairs of angles and one pair of corresponding sides are equal. We know that the sum of the three angles of a triangle is 180° and thus if two pairs of angles are equal, the third pair(180° – sum of equal angles) is also equal. Are the triangles still congruent? You will observe that they are congruent. Suppose, now in two triangles two pairs of angles and one pair of corresponding sides are equal but the side is not included between the corresponding equal pairs of angles. If AB < DE, we can choose a point M on DE such that ME = AB and repeating the arguments as given in Case (ii), we can conclude that AB = DE and so, Δ ABC ≅ Δ DEF. or, BA = ED So, Δ ABC ≅ Δ DEF (by SAS axiom) This is possible only if P coincides with A. Since the triangles are congruent, their corresponding parts will be equal. Thus, Δ PBC ≅ Δ DEF, by the SAS congruence rule. In Δ PBC and Δ DEF, PB = DE (By construction), ∠ B = ∠ E (Given), BC = EF (Given). Let if possible AB > DE and thus, we can take a point P on AB such that PB = DE. 1.Ĭonsider the two triangles, ABC and DEF in which ∠ B = ∠ E, ∠ C = ∠ F, and BC = EFįor proving the congruence of the two triangles three cases arise.ĪB = DE (Assumed), ∠ B = ∠ E (Given), BC = EF (Given). In this lesson, let's learn about the ASA congruence rule in detail. The 5 congruence rules include SSS, SAS, ASA, AAS, and RHS. It is not necessary that all the six corresponding elements of both the triangles must be equal in order to determine their congruency. Thus, we can say that the two triangles are congruent if they are of the same size and same shape.
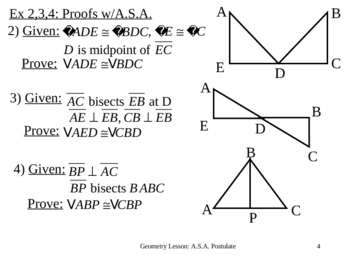
For two triangles to be congruent, the major elements, mainly three angles, and the three sides of a triangle should be equal to the corresponding angles and the corresponding sides of another triangle. ASA congruence rule states that if two angles of one triangle, and the side contained between these two angles, are respectively equal to two angles of another triangle and the side contained between them, then the two triangles are considered to be congruent.


 0 kommentar(er)
0 kommentar(er)
